In this section we look at integrals that involve trig functions In particular we concentrate integrating products of sines and cosines as well as products of secants and tangents We will also briefly look at how to modify the work for products of these trig functions for some quotients of trig functions &=∫(\tan^2x)^2\sec^2x\sec x\tan x\,dx & & \text{Write }\tan^4x=(\tan^2x)^2\\4pt &=∫(\sec^2x−1)^2\sec^2x\sec x\tan x\,dx & & \text{Use }\tan^2x=\sec^2x−1\\4pt &=∫(u^2−1)^2u^2du & & \text{Let }u=\sec x\text{ and }du=\sec x\tan x\,dx\\4pt4 Chapter 10 Techniques of Integration EXAMPLE 1012 Evaluate Z sin6 xdx Use sin2 x = (1 − cos(2x))/2 to rewrite the function Z sin6 xdx = Z (sin2 x)3 dx = Z (1− cos2x)3 8 dx = 1 8 Z 1−3cos2x3cos2 2x− cos3 2xdx Now we have four integrals to evaluate Z 1dx = x and Z

Integrate Tan 2x
Tan^2x formula integration
Tan^2x formula integration-It does not contain any constant of integration Integral of cos^2x We can't just integrate cos^2(x) as it is, so we want to change it into another form, which we can easily do using trig identities Integral of cos^2(2x) Recall the double angle formula cos(2x) = cos^2(x) – sin^2(x) We also know the trig identity sin^2(x) cos^2(x) = 1,Reduction formula for In = ∫tannxdx Let u(x) = tann − 2x v ′ (x) = tan2x Integrating by parts In = (tanx − x)(tann − 2x) − ∫(tan(x) − x)(n − 2)(tann − 3x tann − 1x)dx Let the second integral be J, After expanding and simplifying I get, J = (n − 2)In − 2




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
Sin ( ) 1 cos(2 )x x ii 12 2 cos ( ) 1 cos(2 )x x If there are no sec(x) factors and the power of tan(x) is even and positive, use sec 1 tan22x x to convert one tan2 x to sec2 x Rules for sec(x) and tan(x) also work for csc(x) andIn integral calculus, the Weierstrass substitution or tangent halfangle substitution is a method for evaluating integrals, which converts a rational function of trigonometric functions of into an ordinary rational function of by setting = (/) No generality is lost by taking these to be rational functions of the sine and cosine The general transformation formula isSo the required answer is tanxxc Where c is any constant
If you let u=tanx in integral (tan^2)x you get integral u^2 dx which is not (u^3)/3 c since du= sec^2x dxFormula cos 2 θ = 1 − tan 2 θ 1 tan 2 θ A mathematical identity that expresses the expansion of cosine of double angle in terms of tan squared of angle is called the cosine of double angle identity in tangent•use trigonometric identities to integrate sin2 x, cos2 x, and functions ofthe formsin3x cos4x •integrate products of sines and cosines using a mixture of trigonometric identities and integration by substitution •use trigonometric substitutions to evaluate integrals Contents 1 Introduction 2 2
As there is no way to immediately integrate tan^2 (x) using well known trigonometric integrals and derivatives, it seems like a good idea would be writing tan^2 (x) as sec^2 (x) 1 Now, we can recognise sec^2 (x) as the derivative of tan (x) (you can prove this using the quotient rule and the identity sin^2 (x) cos^2 (x) = 1), while we getSimilarly to the previous examples, this type of integrals can be simplified by the formula \ If the power of the secant \(n\) is even, then using the identity \(1 {\tan ^2}x \) \(= {\sec ^2}x\) the secant function is expressed as the tangent function The factor \({\sec ^2}x\) is separated and used for transformation of the differentialHow to find the integral of tan(2x)In this tutorial we go through the steps to find the integral of tangent(2x) using the usubstitution integration method



How To Integrate X Tan 2 X Dx Quora
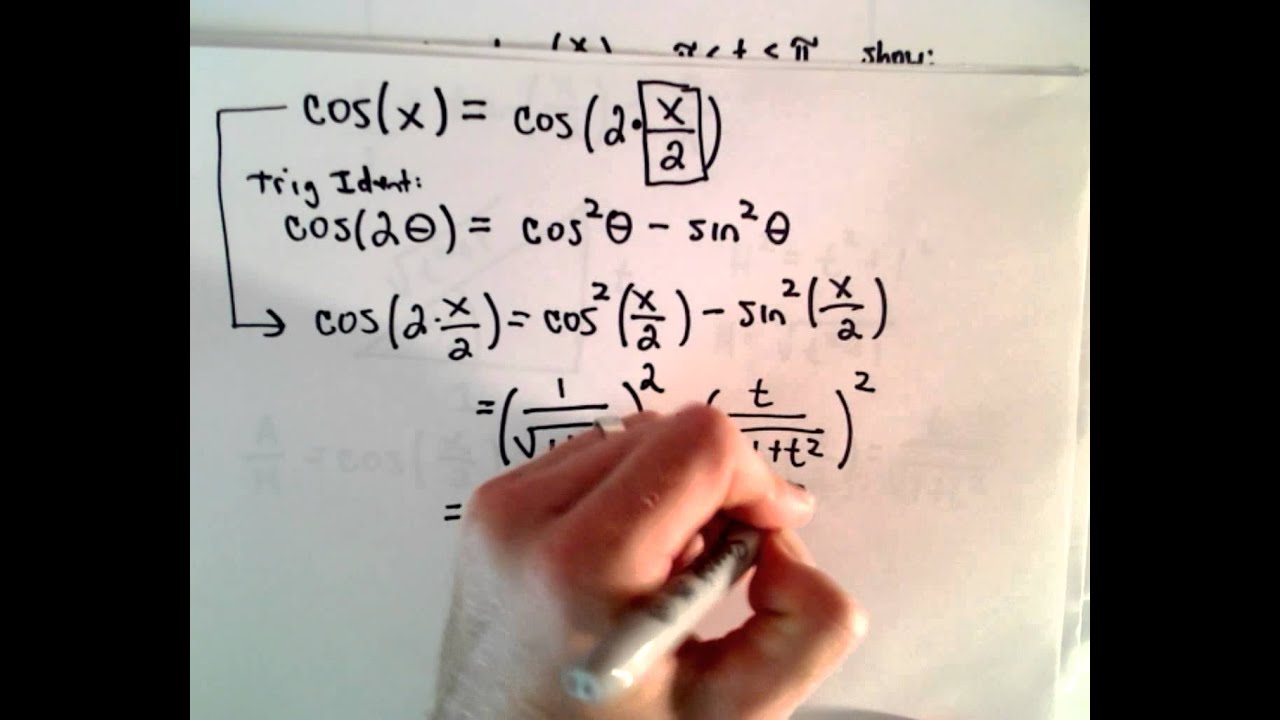



Integrate Rational Function Of Sine And Cosine T Tan X 2 Part 1 Youtube
©05 BE Shapiro Page 3 This document may not be reproduced, posted or published without permission The copyright holder makes no representation about the accuracy, correctness, orIt may be necessary to repeat this process on the tan k − 2 x tan k − 2 x term If k k is even and j j is odd, then use tan 2 x = sec 2 x − 1 tan 2 x = sec 2 x − 1 to express tan k x tan k x in terms of sec x sec x Use integration by parts to integrate odd powers of sec x sec x We know that `tan^2(x)=sec^2(x)1` hence we can rewrite our integral in the following manner `inttan^n(x)dx=inttan^(n2)(x)*tan^2(x)dx=` `inttan^(n2)(x)(sec^2(x)1)dx=`



Use A Change Of Variables Or The Table To Evaluate Chegg Com
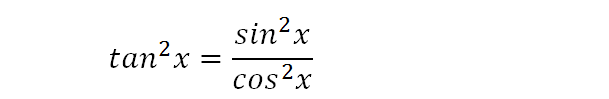



Integrate Tan 2x By Parts
SOLUTION We could evaluate this integral using the reduction formula for (Equation 567) together with Example 3 (as in Exercise 33 in Section 56), but a better method is to write and use a halfangle formula tan x tan2x sec2x 1 tan2x y tan3x dx y sec x dx ln sec x tan x C∫ sec 2 x dx = tan x C;The reduction formulae can be extended to a range of functions The procedure, however, is not the same for every function Eg1 Find a reduction formula to integrate ∫sin n x dx and hence find ∫sin 4 x dx ∫sin n x dx = ∫sin n1 x sin x dx Let u = ∫sin n1 x and dv/dx = sin x So, du/dx = (n1)sin n2 x cos x;




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo



What Is Math Int Tan 2 2x Dx Math Quora
To integrate tan2x, also written as ∫tan2x dx, and tan 2x, we use the u substitution because the integral of tanu is a standard solution in formula books Let u=2x Then, du/dx=2 We transpose for dx to get the above expression Hence, our new integration can be writtin in terms of u and is simpler to solveSo tan^2(x)=sec^2(x)1;integrate this equation on both sides w r to x We know that integration of sec^2(x) is tanx c;Current Location > Math Formulas > Calculus > Integrals of Trigonometric Functions Integrals of Trigonometric Functions Don't forget to try our free app Agile Log sec 2 x tan x c csc 2 xcot x c Example 1 Calculate the following integral ∫x 2 sin x 3 dx Solution




How Do You Integrate 1 Tan2x Sec2x Dx Socratic




Misc 44 Mcq Value Fo Tan 1 2x 1 1 X X2 Dx Is
These integral formulas are equally important as differentiation formulas Some other important integration formulas are Classification of Integral Formulas A) Integral product of two functions = first function × integral of the second function – integral of {differential coefficient of the first function × integral of the second function} ∫ f1(x) f2(x) = f1(x)∫ f2(x)dx − ∫ d dxf1(x) ∫ f2(x)dxdx B) ∫ exf(x) f ′ (x)dx = ∫ exf(x)dx CTo integrate tan^22x, also written as ∫tan 2 2x dx, tan squared 2x, (tan2x)^2, and tan^2(2x), we start by utilising standard trig identities to change the form of the integral Our goal is to have sec 2 2x in the new form because there is a standard integration solution for that in formula booklets that we can use We recall the Pythagorean trig identity, and multiply the angles by 2 throughout




Can I Integrate Math Tan 2 X Sec 3 X Math Using The Substitution Quora



1
`int ((3 ln\ 2x)^3)/(x) dx` Let u = 3 ln 2x We can expand out the log term on the right hand side as follows 3 ln 2x = 3 ln 2 ln x Now the first 2 terms on the right are constants (whose derivative equals zero) and the derivative of the natural log of x is `1/x` Then `du = 1/x dx`598 integration techniques If the exponent of cosine is odd, split off one cos(x) and use the identity cos2(x) = 1 sin2(x) to rewrite the remaining even power of cosine in terms of sine Then use the change of variable u = sin(x) If both exponents are even, use the identities sin2(x) = 1 2 1 2 cos(2x) and cos2(x) = 1 2 1 2 cos(2x) to rewrite the integral in terms of powers The integral of $$\int\tan^n(x)dx$$ I found this proof online for the answer and it went like this $$\int\tan^{n2}(x)\tan^2(x)dx$$ $$\int\tan^{n2}(x)(\sec^2(x)1)dx$$ $$\int\tan^{n2}(x)\sec^2(x)dx\int\tan^{n2}(x)dx$$ Then using usubstitution it yielded the reduction formula $$\frac{\tan^{n1}(x)}{n1}\int\tan^{n2}dx$$




Integration Calculus Notes




Integrate Tan2x
$\int ( \sec^2 x) = \tan x C$ $\int (\csc^2 x) = \cot^2 x C$ $\int ( \sec (x) \tan (x) )=\sec x C$ $\int (\csc (x) \cot (x)) = \csc x C$ $ \int (\tan x) = ln \sec x C$ $ \int (\cot x) = ln \sin x C$ $ \int (\sec x) = ln \sec x \tan x C$ $ \int (\csc x) = ln \csc x – \cot x C$ Integration formulas Related to Inverse Trigonometric FunctionsClick here👆to get an answer to your question ️ Integrate the following tanxtan2xtan3x = Figure 711 To find the area of the shaded region, we have to use integration by parts For this integral, let's choose u = tan − 1x and dv = dx, thereby making du = 1 x2 1 dx and v = x After applying the integrationbyparts formula (Equation 712) we obtain Area = xtan − 1x1 0 − ∫1 0 x x2 1 dx




Integral Of The Secant Function Wikipedia




Integral Of Secant Cubed Wikipedia
Chapter 7 Class 12 Integration Formula Sheet by teachoocom Basic Formulae = ^( 1)/( 1) , 1 , = = sin x C = cos x C 2 = tan x c 2 = cot x c = sec x c∫ a x dx = (a x /ln a) C ; u= tan^3 (x) dv=1 du= 3tan 2 (x)sec 2 (x)dx v = x = xtan 3 ∫x3tan 2 (x)sec 2 (x)dx again, ∫x3tan 2 (x)sec 2 (x)dx u = x dv= tan 3 sec 2 du = dx v = (tan ^4 )/¼ This is going no where to what the reduction formula is supposed to look like
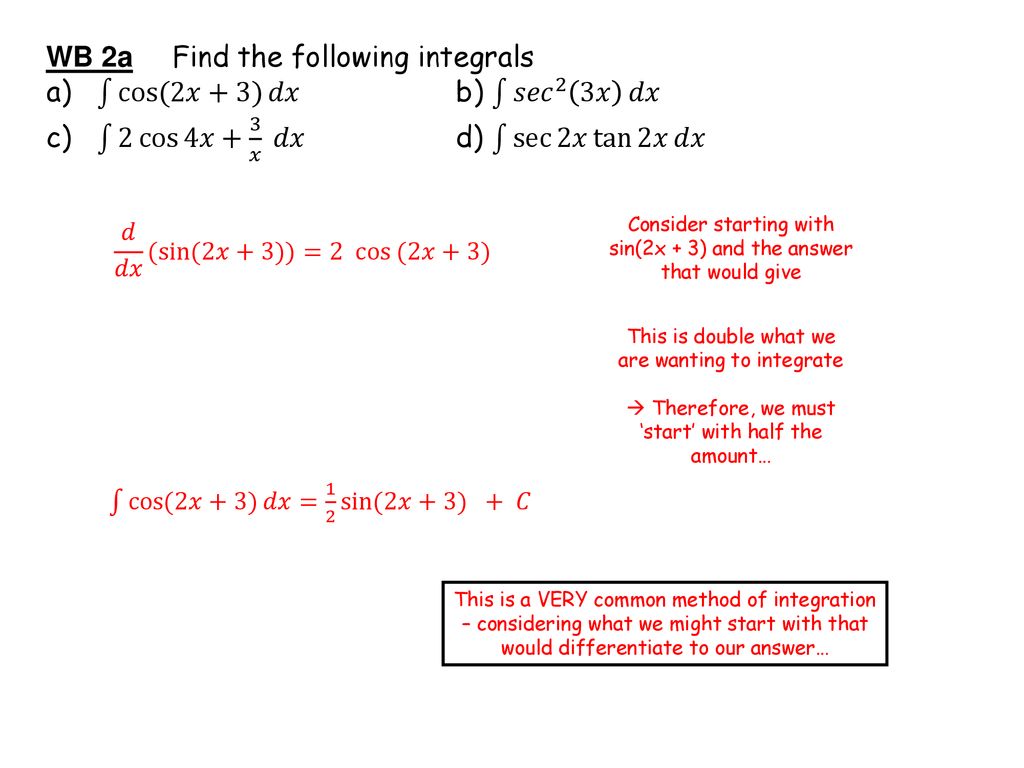



Integration Trig Identities Ppt Download




Integral Of Sec 2 X Sqrt 1 Tan 2 X Using The Arcsine Function Math Videos Maths Exam Calculus
In trigonometry, tangent halfangle formulas relate the tangent of half of an angle to trigonometric functions of the entire angle The tangent of half an angle is the stereographic projection of the circle onto a line Among these formulas If u still do not understand follow these procedures 1) Use tan (ab) to bring the entire integral in terms of tanx alone Note you might have to use tan2x = 2tanx/sec^2x 2)After you have got an expression in terms of tanx integral { (4tan^3x)/ (sec^2x (Sec^2x2tan^2x)) (2tan^3x)/ (sec^2x2tan^2x)}Solve the integral = ln u C substitute back u=cos x = ln cos x C QED 2 Alternate Form of Result tan x dx = ln cos x C = ln (cos x)1 C = ln sec x C Therefore
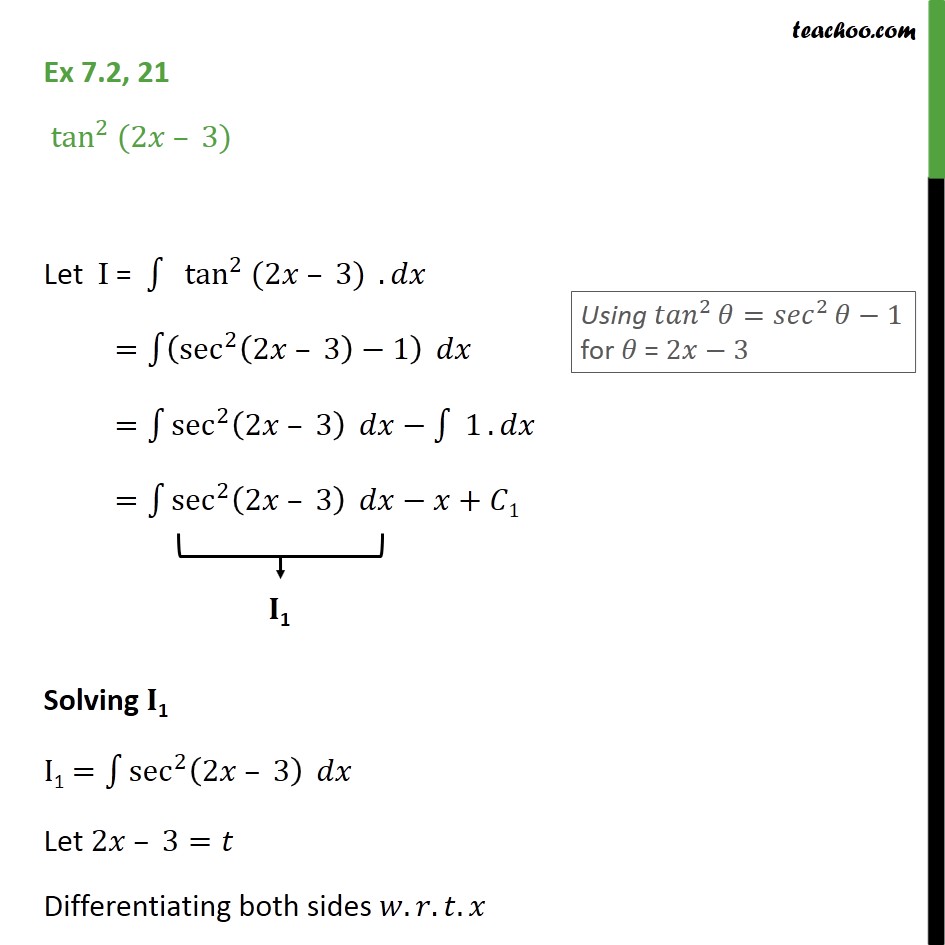



Ex 7 2 21 Integrate Tan2 2x 3 Class 12 Cbse Ex 7 2
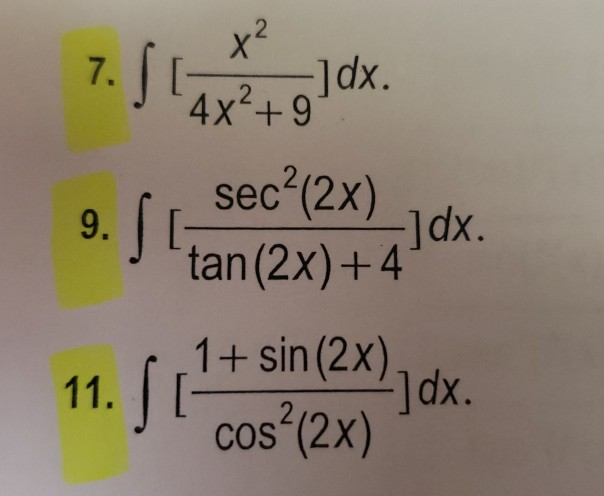



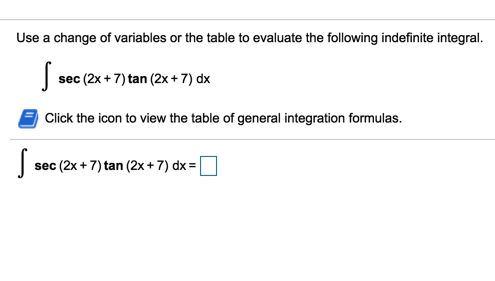
Solved Use Integration Formulas Along With Any Algebraic Chegg Com
Integration of Cos2x The integral of cos2x = (1/2)sin(2x) C, Where C is constant Finding the integral of Cos (2x) f (x) = cos (x) g (x) = 2x f (g (x))= cos (2x) Let u = g (x) =2x then du = g' (x) = 2dx, and d (x) = (1/2)du Plug in u for 2x and (1/2)du for dx∫ (1/x) dx = ln x C; You can start by writing tan^2(x)=sin^2(x)/cos^2(x) giving inttan^2(x)dx=intsin^2(x)/cos^2(x)dx= using sin^2(x)=1cos^2(x) you get =int(1cos^2(x))/(cos^2(x))dx=int1/cos^2(x)1dx= =int1/cos^2(x)dxint1dx= =tan(x)xc
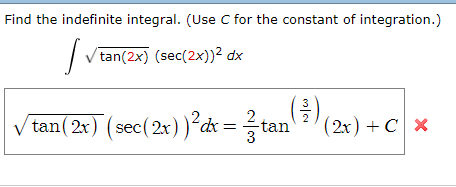



Find The Indefinite Integral Use C For The Constant Chegg Com



Integral Of Sec 6 X Tan 2 X Dx
1 Integration By Substitution (Change of Variables) We can think of integration by substitution as the counterpart of the chain rule for di erentiation Suppose that g(x) is a di erentiable function and f is continuous on the range of g Integration by substitution is given by the following formulas Inde nite Integral Version Z f(g(x))g0(x)dx= ZTo find some integrals we can use the reduction formulas These formulas enable us to reduce the degree of the integrand and calculate the integrals in a finite number of steps Below are the reduction formulas for integrals involving the most common functions ∫xnemxdx = 1 m xnemx − n m ∫xn−1emxdx ∫ emx xn dx = − emx (n−1)xn−1 Introduction to Integral formulas The list of integral calculus formula is here with all the rules which are needed to solve integration The formula sheet of integration include basic integral formulas, integration by parts and partial fraction, area as a sum and properties of definite integralAt first take a look at indefinite integration




Tan 2x Formula What Is Tan 2x Formula Examples
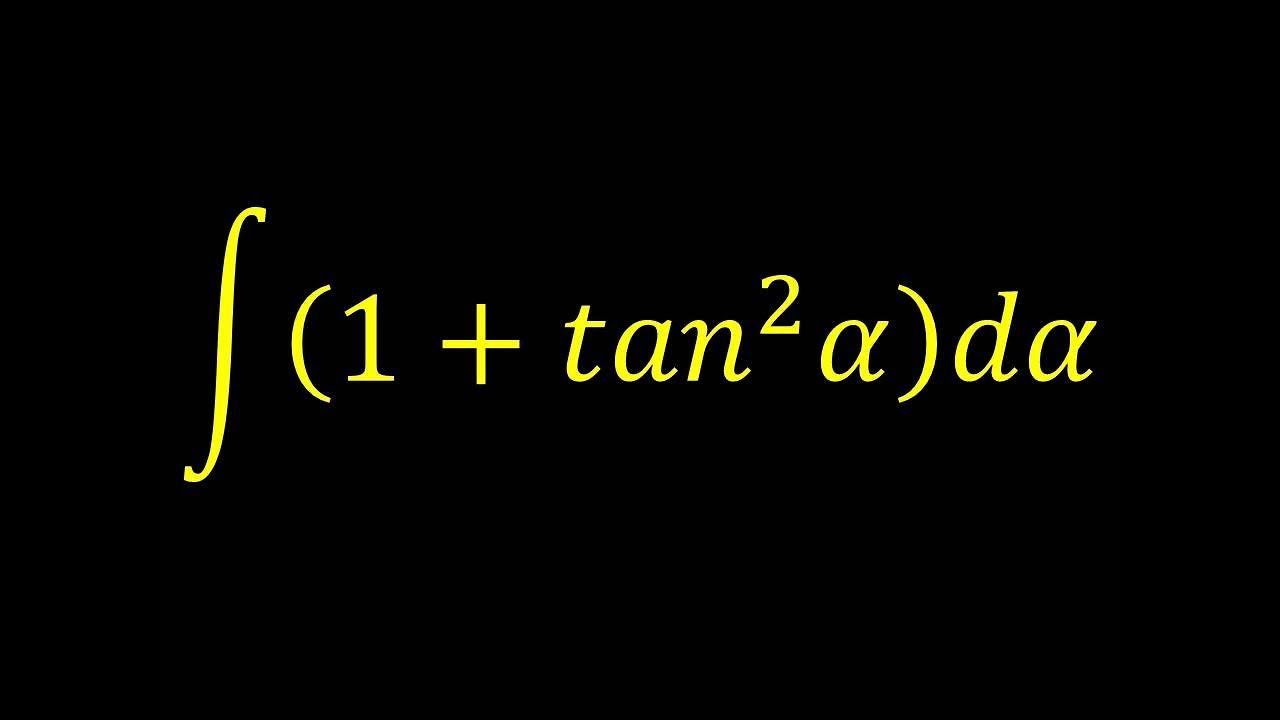



Integral Of 1 Tan 2 X Integral Example Youtube
Ln ln 2 2 tan(x a dx x x a x a2 2 2 2 1) ( ) x a ∫ = − − sin ln sin ln cos ln( ) ( )( ) ( ) 2 x ∫ x dx x x= − cos ln sin ln cos ln( ) ( )( ) ( ) 2 x ∫ x dx x x=∫ sec x (tan x) dx = sec x C;∫ e x dx = e x C;




Tan2x ただの悪魔の画像




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
Integrals (inverse functions) Derivatives; Ex 74, 9 sec 2 tan 2 4 Let tan = Diff both sides wrt x sec 2 = = sec 2 Integrating the function sec 2 tan 2 4 Putting value of tan = and = sec 2 = sec 2 tC being a constant So integration of tan^2(x) would be tanx x c;




Ex 7 2 21 Integrate Tan2 2x 3 Class 12 Cbse Ex 7 2



F4ntussz Z2tvm
Integral of u^2 is NOT (u^3)/3 c Rather, integral of (u^2)du = (u^3)/3 c In (tan^2)x your 1st mistake is not writing dx Note that dx is NOT always du!!!!!Substitution\\int\frac {e^ {x}} {e^ {x}e^ {x}}dx,\u=e^ {x} integralcalculator \int\tan^ {2} (x)dx en Sign In Sign in with Office365 Sign in with Facebook OR∫ csc x ( cot x) dx = – csc x C;




Integrate Tan 2x By Parts




Qprove That Tan X 2 Tan 2x 4 Tan 4x 8 Cot 8x Cot X Mathematics Topperlearning Com Blpusg55
Introduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formula Here is a summary for this final type of trig substitution √a2b2x2 ⇒ x = a b tanθ, −π 2 < θ < π 2 a 2 b 2 x 2 ⇒ x = a b tan θ, − π 2 < θ < π 2 Before proceeding with some more examples let's discuss just how we knew to use the substitutions thatIntegration Reduction Formulas Any positive integer power of sin x can be integrated by using a reduction formula Example Prove that for any integer n 2, Z sin n xdx= 1 n sin C to find the integrals of tan2 x, tan3 x, tan4 x and tan5 x Using the identity tan2 x =sec2 x 1, Z tan n xdx= Z tan n2 xtan2xdx= Z tan n2 x(sec2x 1) dx = Z tan




1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube
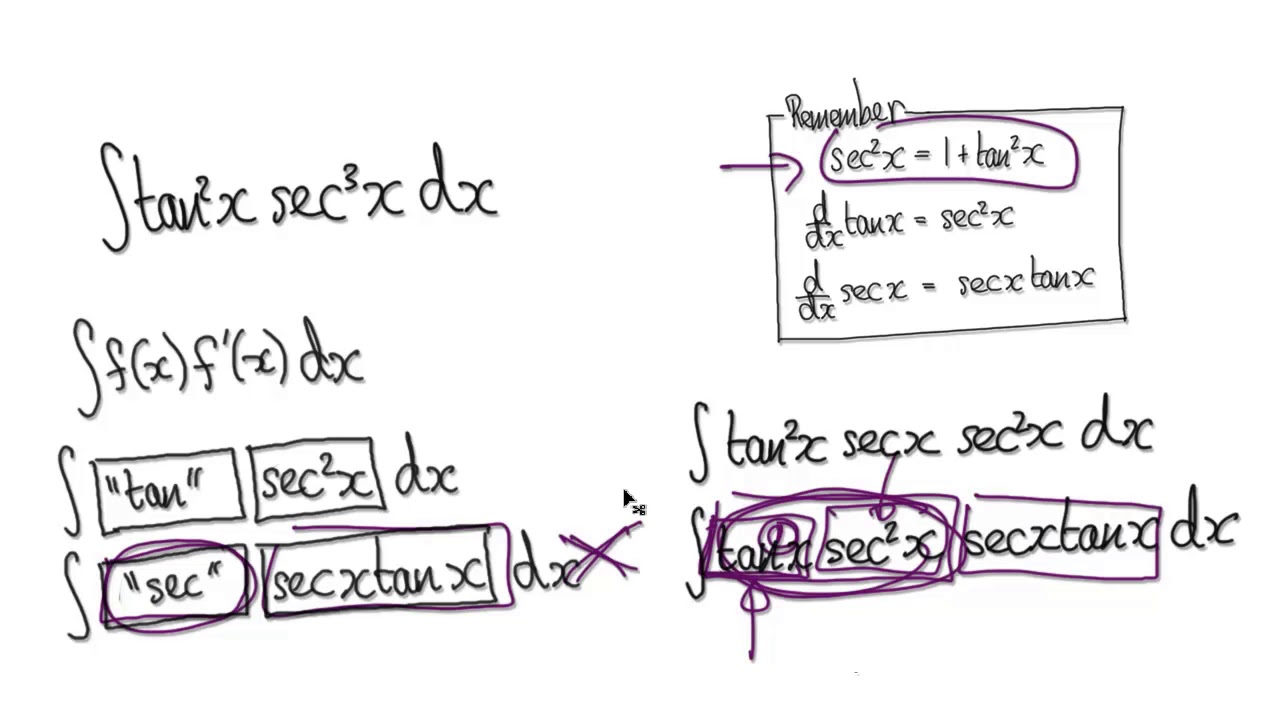



Video 34 6 Integrate Tan 2x Sec 3x Reduction Formula Practice Youtube
∫ csc 2 x dx = cot x C;V = cos x




Integrate Sec 2x Method 2




Unit 4 Integration B C A Study




Weierstrass Substitution Wikipedia
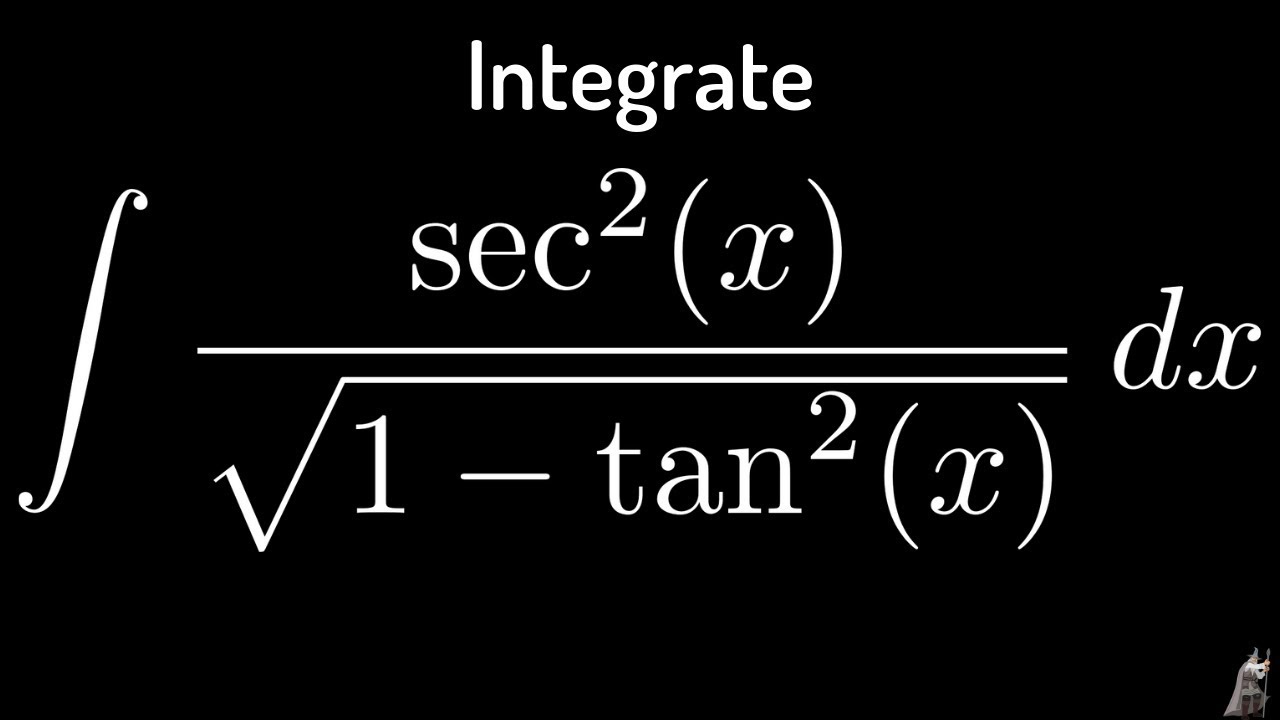



Integral Of Sec 2 X Sqrt 1 Tan 2 X Using The Arcsine Function Youtube
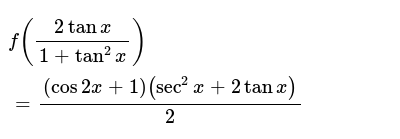



F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2



Integrals Of Trigonometric Functions Web Formulas




Evaluate Inttan X Theta Tan X Theta Tan2x Dx
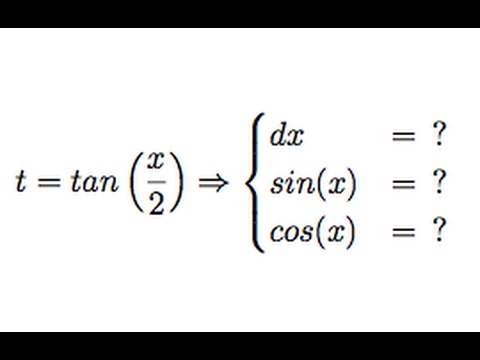



T Tan X 2 Weierstrass Substitution Youtube




Integral Of Tan2x Integration Of Tan2x Antiderivative Of Tan2x Integral Of Tan 2x Youtube
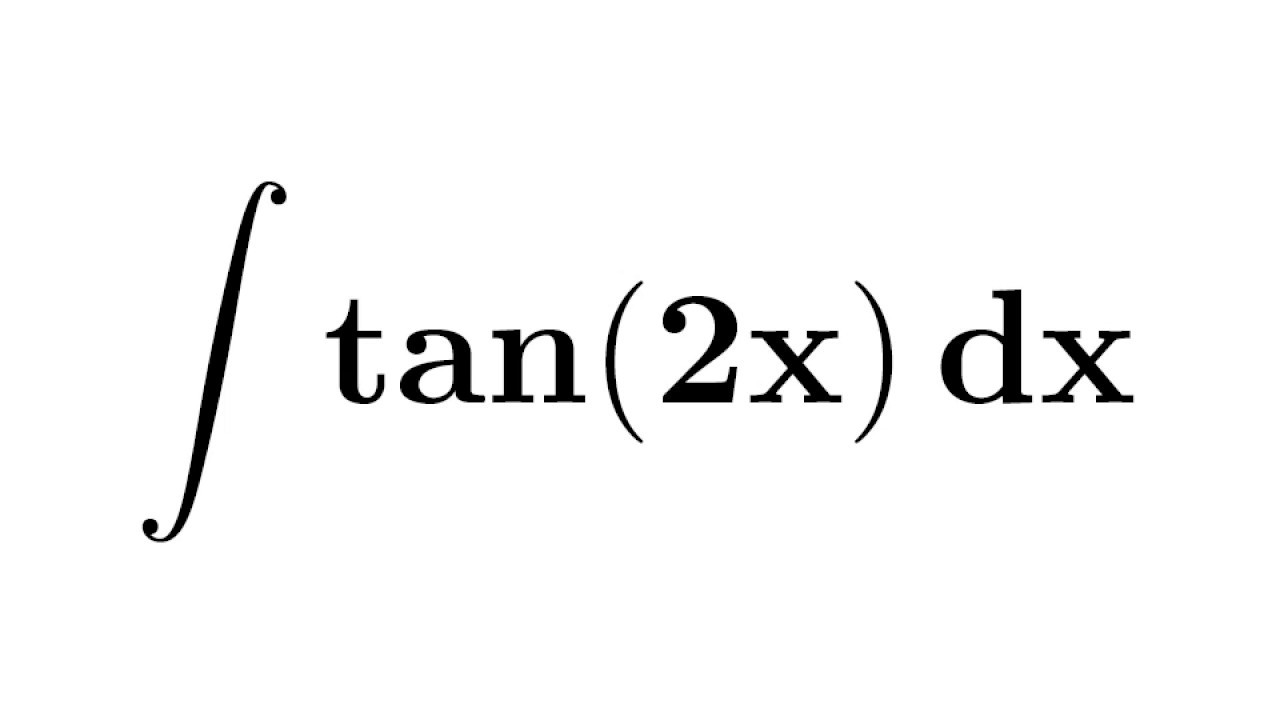



Integral Of Tan2x Integration Of Tan2x Antiderivative Of Tan2x Integral Of Tan 2x Youtube




Evaluating Trig Integral Int Tan 3x Sec 2x Dx Mathematics Stack Exchange




Tan2x ただの悪魔の画像




Integral 1 Tan 2 X Sec 2 X Youtube




Integrate Tan 2x




Solving The Integral Of Cos X Video Lesson Transcript Study Com



bestpictjcry Tan 2x Tan 2x




Integral Of Sec 2x Tan 2x Integral Example Youtube




Weierstrass Substitution Wikipedia




Int 1 Tan 2x 1 Tan 2x Dx




Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube




What Is The Integration Of Tan 2x Solution Quora



What Is The Integral Of Tan X 2 Quora




Analytic Trig Ppt Video Online Download
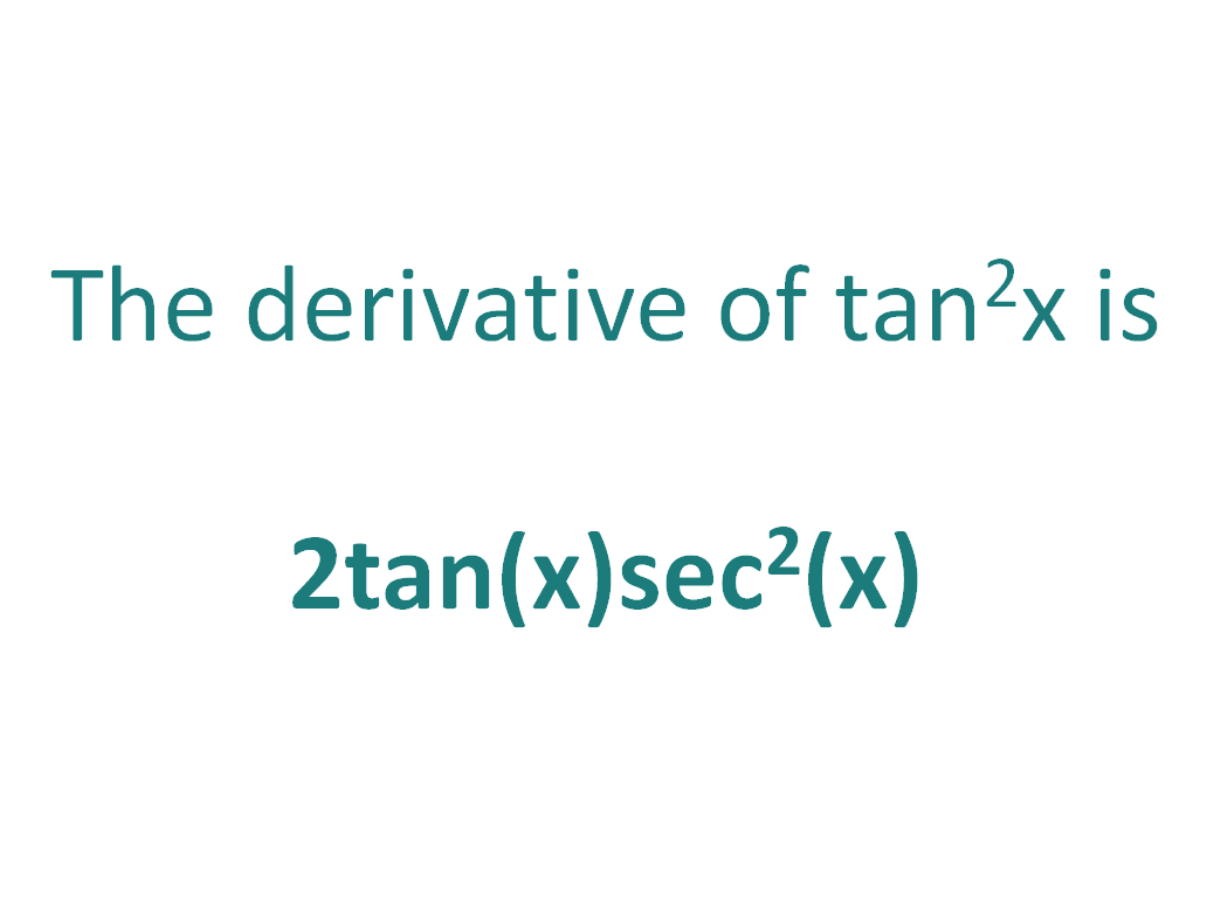



The Derivative Of Tan 2x Derivativeit
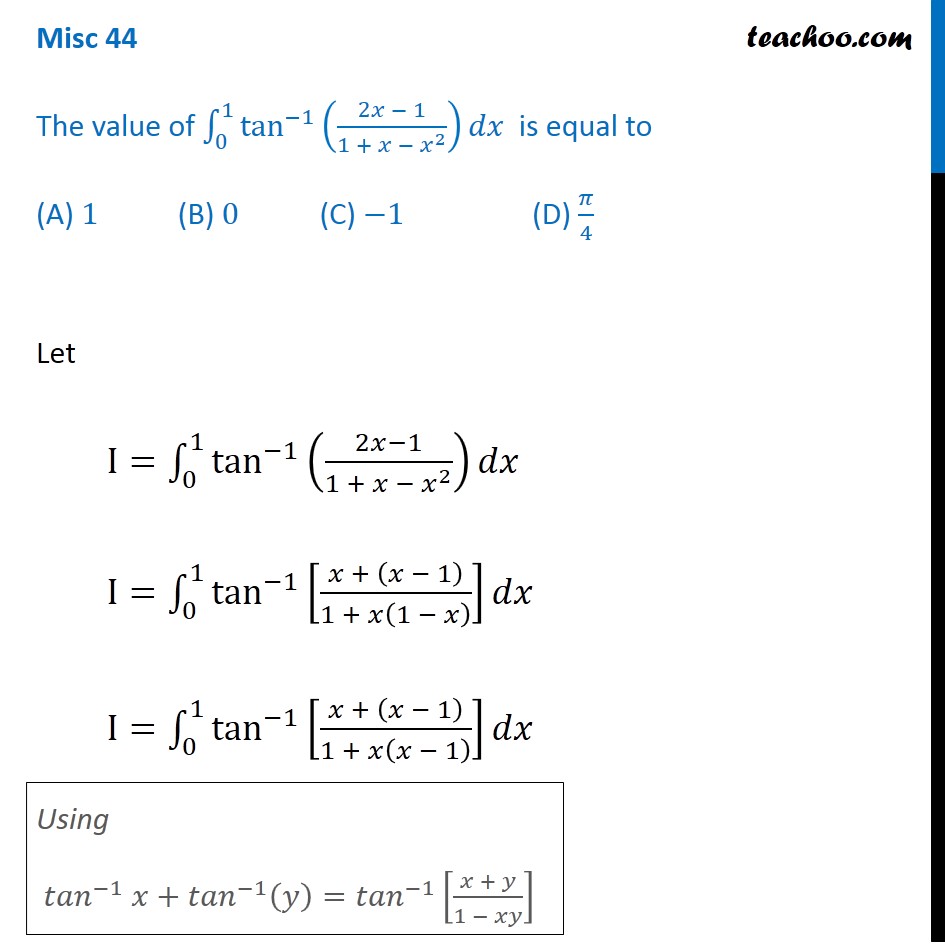



Misc 44 Mcq Value Fo Tan 1 2x 1 1 X X2 Dx Is




Integral Sec 2 2x 2 Dx A Tan 2x 2 2 C Chegg Com



How Do I Integrate Tan 2 X Youtube



What Is The Formula Of Tan2x Quora
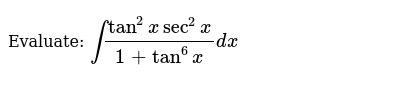



Evaluate Int Tan 2xsec 2x 1 Tan 6x Dx
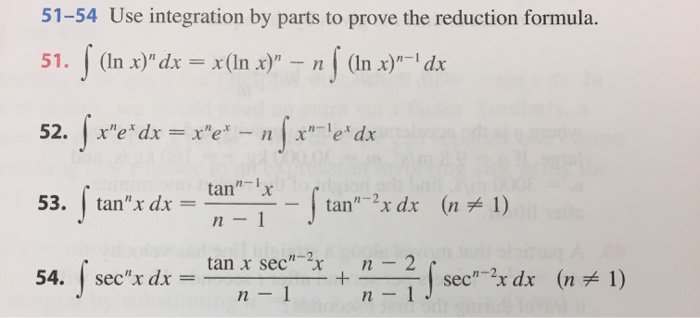



Use Integration By Parts To Prove The Reduction Chegg Com




The Integral Int Sec 2x Secx Tanx 9 2 Dx Equals For Some Arbitrary Constant K Dot 1 Secx Tanx 11 2 1 11 1 7 Secx Tanx 2 K 1 Secx Tanx 1 11 1 11 1 7 Secx Tanx 2 K 1 Secx Tanx 11 2 1 11 1 7 Secx Tanx 2 K 1



1




Powers Of Trigonometric Functions




Integration Calculus Notes



Integral Of Sec 6 X Tan 2 X Dx




Integrate W R T X Tan3x Tan2x Tan5x




Integration Calculus Notes
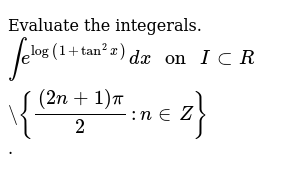



Evaluate The Integerals Br Int E Log 1 Tan 2 X Dx



1



Tan2x ただの悪魔の画像




Integral Of Tan 2 X Sec X Youtube



1




Integrate Sec 2x Method 1




Integrate Tan 2x By Parts
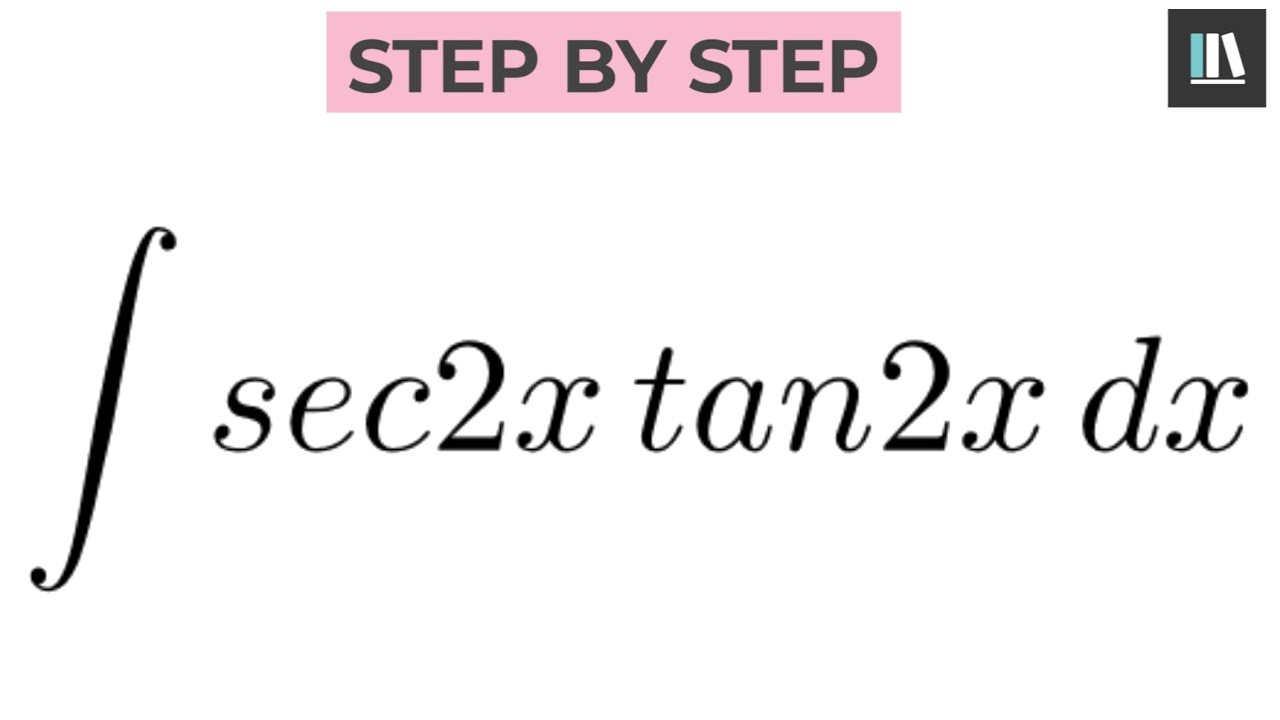



Integral Sec2x Tan2x Dx Youtube




Ex 7 6 24 Mcq Integrate E X Sec X 1 Tan X Dx Is A E X Cos X



What Is The Integration Of Tanx Tan2x Tan3x Dx Quora




Integral Of Tanx Tan2x Tan3x Dx Youtube



What Is The Integration Of Tanx Tan2x Tan3x Dx Quora




Integrating Tan 2 2x Youtube
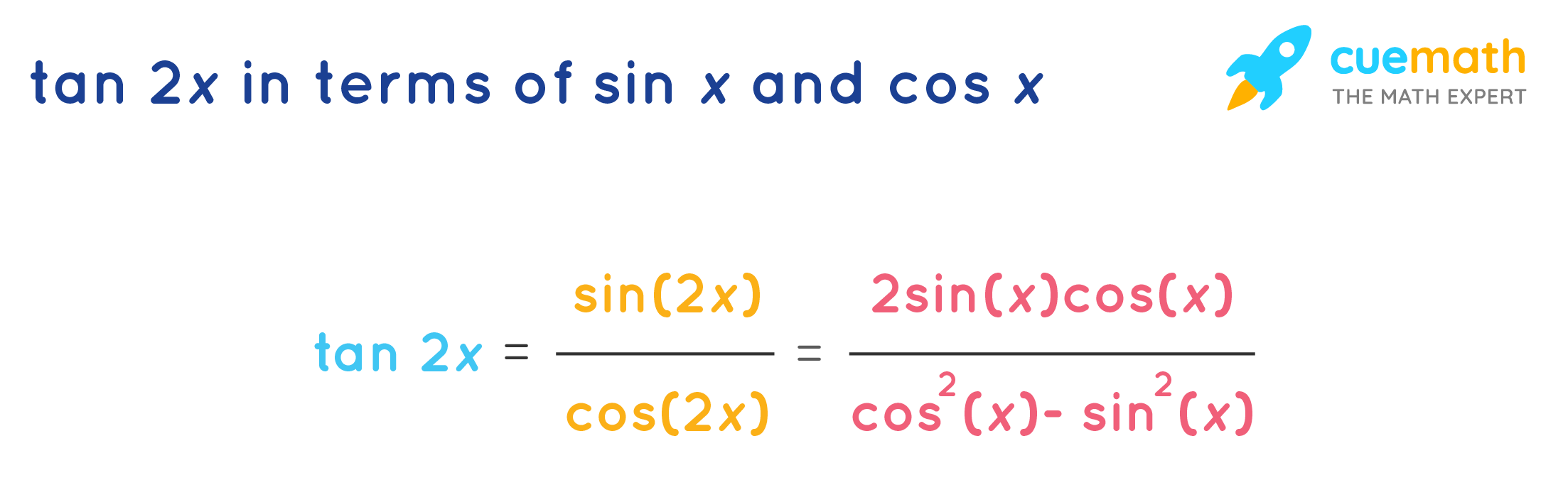



Tan 2x Formula What Is Tan 2x Formula Examples




Integration Of Tan 2 X Sec 2 X Youtube
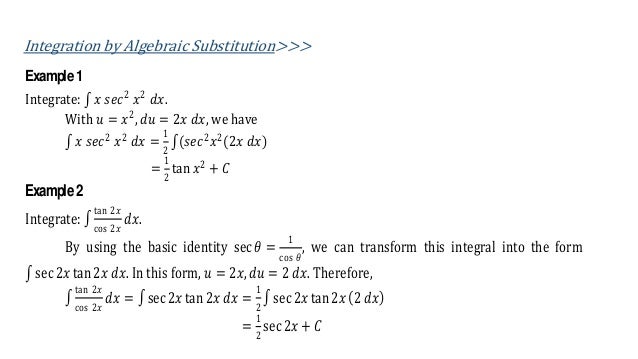



Integration Of Trigonometric Functions




Integral Of Tan 2x Cot 2x 2 Calculus 1 Trig Integrals Youtube




Integral Of Tan 2x Youtube
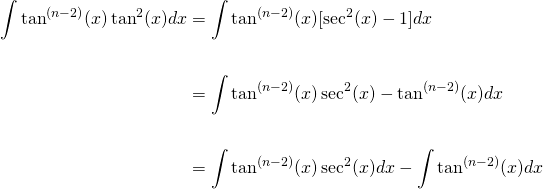



Reduction Formula For The Integral Of Tan N X Dx Steemit




Weierstrass Substitution Wikipedia




Integration Calculus 2




Integral Of Tan X Video Khan Academy




Integral Of Secant Cubed Wikipedia




Integral Tan 2 X Sec X Youtube




Integrate Cosec 2x



How To Integrate Tan 2x Youtube




Examples Int Tan 2x Sec 4x Dx




What Is Integral Of Sin2x Tan2x Quora




How To Integrate Math Int Sin 4x Cdot E Tan 2x Dx Math Quora
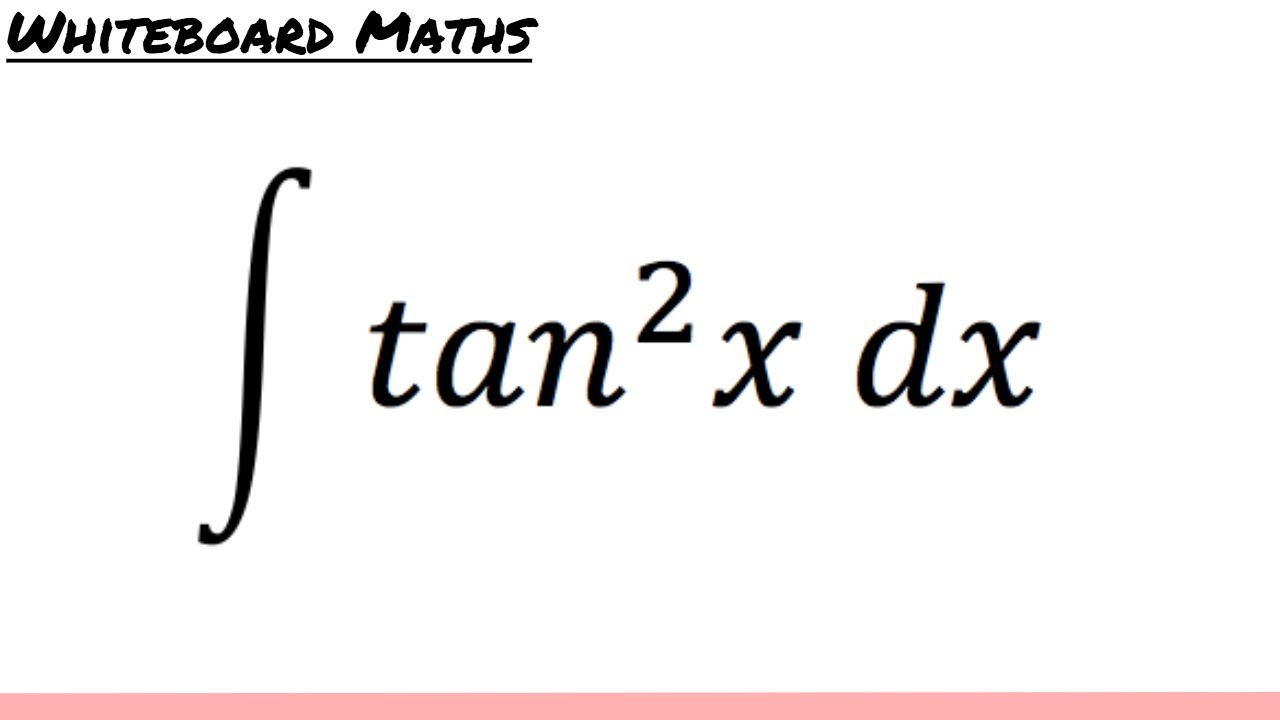



Integral Of Tan 2 X Youtube
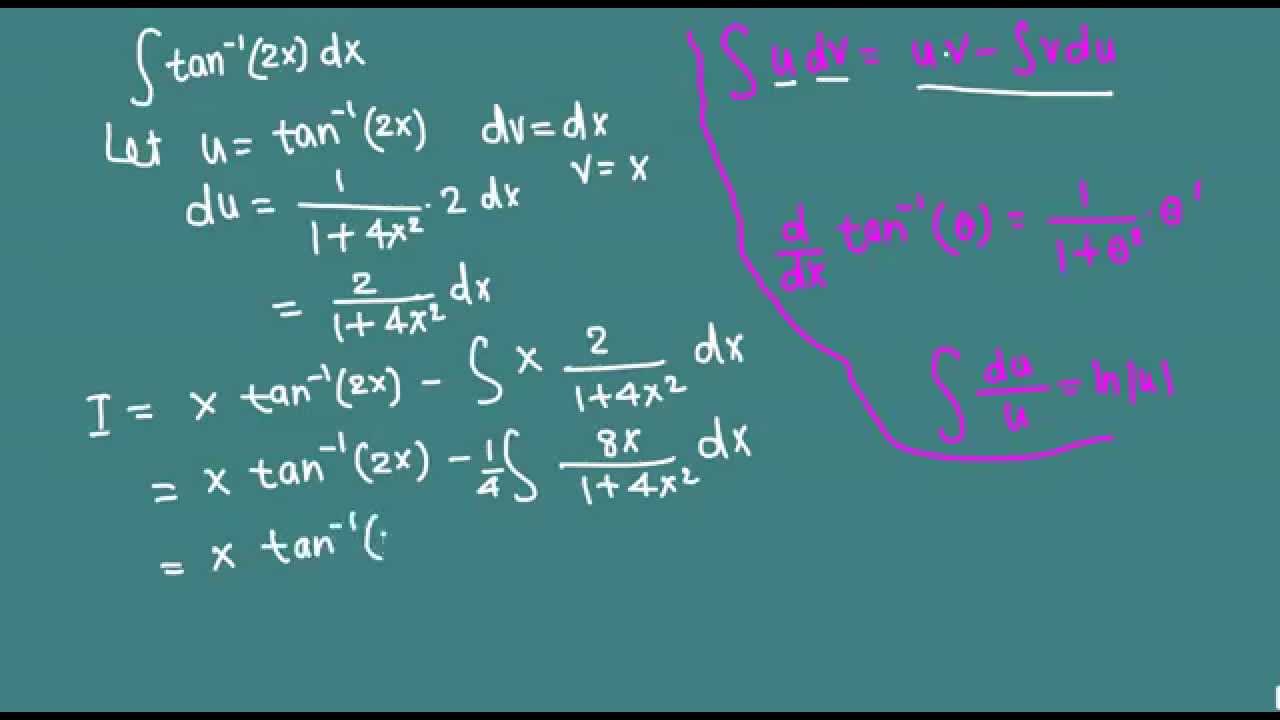



Integration Of Inverse Tan 2x Integration By Parts Youtube




Weierstrass Substitution Wikipedia



What Is The Integral Of Cotx Quora




Weierstrass Substitution Wikipedia




Integral Of The Secant Function Wikipedia
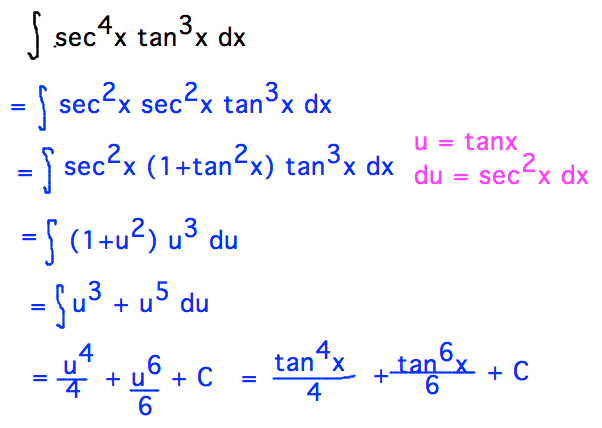



Geneseo Math 222 01 Trigonometric Integration




Weierstrass Substitution Wikipedia



0 件のコメント:
コメントを投稿